What is Node, Branch, Loop and Mesh in an Electric Circuit, and How to Identify them?
Understanding the fundamentals of electric circuits and networks is crucial for solving and analyzing complex systems using network theorems. Four key elements and terms in this process are Nodes, Branches, Loops, and Meshes.
In this tutorial, we will explore the roles of Nodes, Branches, Loops, and Meshes in electrical circuits, followed by methods to determine their quantities. This knowledge enables us to apply circuit-solving techniques to simplify complex networks.
Let’s break down these concepts step by step.
First, we’ll consider a simple electric circuit illustrated in fig-1, comprising 7 components.
Node
A point or junction where two or more elements of a circuit (such as resistors, capacitors, inductors, etc.) meet is called a Node. In other words, a Node is a point of connection between two or more branches.
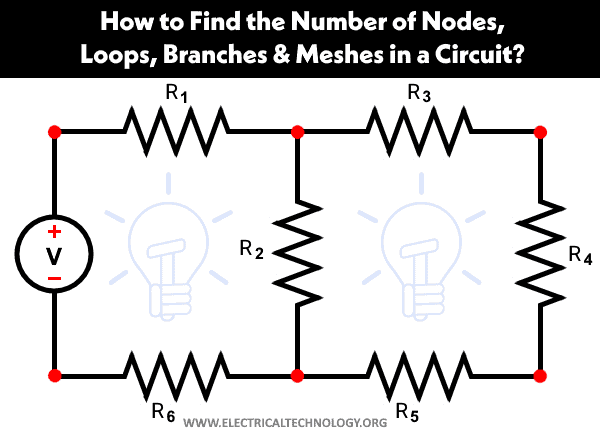
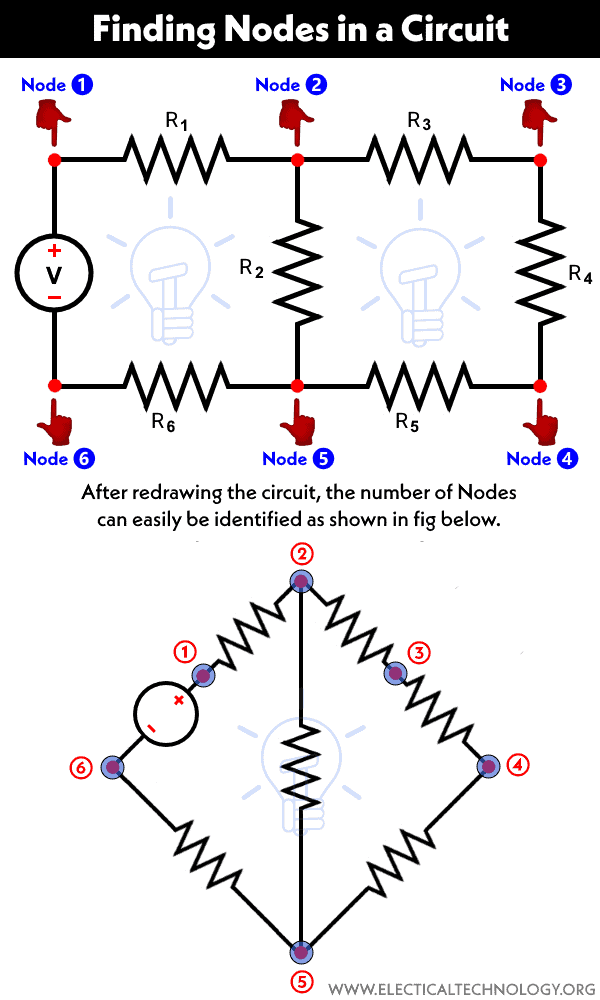
Finding Nodes in an Electric Circuit
After redrawing the circuit shown in fig-1 above, we obtain the equivalent circuit shown below. Now, let’s determine the number of nodes. As illustrated in fig-2, there are 6 nodes.
Branch
A part or section of a circuit located between two junctions is called a branch. Within a branch, one or more elements with two terminals can be connected. In other words, it may consist of any two terminals connected by a single component, such as a voltage source, current source, resistor, etc.
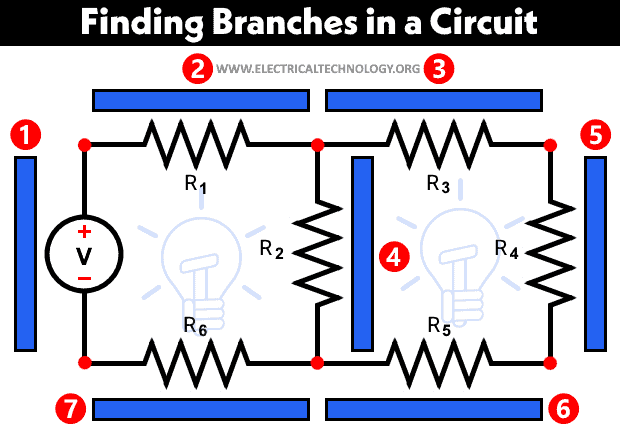
Finding Branches in an Electric Circuit
The circuit shown in figure-3 consists of seven branches, which include a voltage source denoted by “V” and six resistors. Each branch is represented by a blue-colored horizontal bar over it.
Loop
A closed path within a circuit containing more than two meshes is referred to as a loop. In other words, a loop can encompass multiple meshes, but a single mesh cannot contain more than one loop. Put simply, a mesh represents a closed path within a circuit.
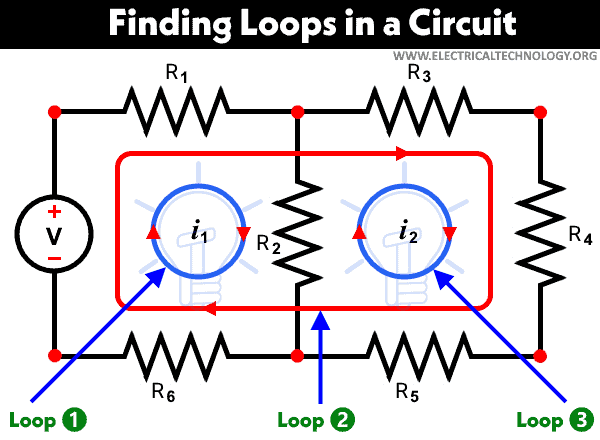
Finding Loops in an Electric Circuit
The number of loops in a circuit can be determined using the fundamental theorem of circuit and network topology.
l = b – n + 1
Where:
- l = Number of Loops
- b = Number of branches
- n = Number of nodes
Good to Know: The formula “L = B – N + 1” is used to find the number of independent loops, which in this case is 2. Loop 1 and Loop 3 are independent, while Loop 3 depends on both Loop 1 and Loop 2.
Therefore, there are 2 independent loops, and the total number of loops is 3 in fig-4.
Mesh
A closed loop that doesn’t contain any other loop within it is referred to as a mesh. In other words, any path within a circuit that doesn’t include any other path is considered a mesh.
Finding Meshes in an Electric Circuit

Using this definition, there are two meshes in the circuit illustrated in fig-5.
Good to Know: A loop can be a mesh, but a mesh cannot be a loop.
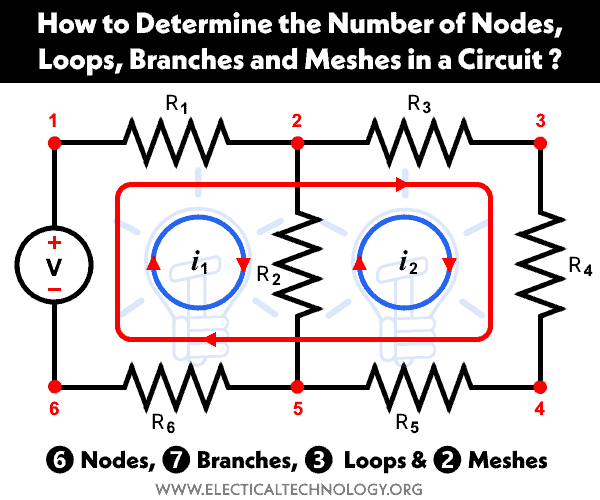
The overall circuit depicted in fig-1 comprises 6 Nodes, 7 Branches, 3 Loops, and 2 Meshes, as illustrated in fig-6.
Related Posts:
- What is an Electric Circuit? Types of Circuits, Network & Parts Of Circuit
- The Main Difference between Linear and Nonlinear Circuit
- Difference between Unilateral and Bi-Lateral Circuits & Elements
- Difference between Active and passive Components
- Difference Between Current and Voltage
- Difference between AC and DC (Current & Voltage)
- What is Electric Current, its Unit, Formula, Types & Applications
- What is Voltage? its Unit, Formula, Types & Applications
- What is Resistance? Resistivity (ρ) & Specific Resistance Ω.
- What is Electrical Power? Types of Electric Power and their Units
- What is Electrical Energy? Its Unit, Formula & Applications
- What is Electricity? Types, Sources & Generation of Electricity
- What is Volt (V)? Unit in Electrical Engineering and Physics
- What is Ampere (A)? Unit in Electrical Engineering & Physics
 Why Does an Electric Tester Not Work in DC Circuits?
Why Does an Electric Tester Not Work in DC Circuits? Why Do The Positive And Negative Wires Spark When Touched?
Why Do The Positive And Negative Wires Spark When Touched? What are the Cuts on the Rotor of Motor’s Armature?
What are the Cuts on the Rotor of Motor’s Armature? Why Do Wind Turbines Have 3 Blades Instead of 2 or 5?
Why Do Wind Turbines Have 3 Blades Instead of 2 or 5? Difference Between Edge Triggering and Level Triggering
Difference Between Edge Triggering and Level Triggering Difference between Zener Diode and Avalanche Diode
Difference between Zener Diode and Avalanche Diode